KEAM 2024 Question Paper with Answer Key (June 9)
KEAM 2024 Question Paper (June 9) is available for download here. Office of The Commissioner for Entrance Examinations (CEE Kerala) conducted KEAM Engineering exam 2024 in CBT mode on June 9 in afternoon shift from 2 PM to 5 PM. KEAM Engineering 2024 Question Paper consists total of 150 questions carrying 4 mark each with negative marking of 1 for each incorrect answer. KEAM 2024 Question Paper includes Mathematics with 75 questions, Physics with 45 questions and Chemistry with 30 questions to be attempted in total of 180 minutes.
KEAM 2024 Question Paper with Answer Key PDF (June 9)
| KEAM 2024 (June 9) Question Paper with Answer Key | Check Solution |
If the displacement of a body moving on a horizontal surface is 151.25 cm in a time interval of 2.25 s, then the velocity of the body in the correct number of significant figures in cm s\(^{-1}\) is:
View Solution
The dimensions of the torque is:
View Solution
A particle is projected at an angle \( \theta \) with the x axis in the xy plane with a velocity \( \mathbf{v} = 6\hat{i} - 4\hat{j} \). The velocity of the body on reaching the x axis again is:
View Solution
The displacement (x) – time (t) graph for the motion of a body is a straight line making an angle 45\(^{\circ}\) with the time axis. Then the body is moving with:
View Solution
A ball is thrown up vertically at a speed of 6.0 m/s. The maximum height reached by the ball (Take \( g = 10 m/s^2 \)) is:
View Solution
The INCORRECT statement is:
View Solution
A bullet of 10 g, moving at 250 m/s, penetrates 5 cm into a tree limb before coming to rest. Assuming uniform force being exerted by the tree limb, the magnitude of the force is:
View Solution
A block of mass \( M \) is kept on the floor of a lift at the centre. The acceleration with which the lift should descend so that the block exerts a force of \( \frac{Mg}{4} \) on the floor of the lift is:
View Solution
A particle of mass 40 g executes simple harmonic motion of amplitude 2.0 cm. If the time period of oscillation is \( \frac{\pi}{20} \) s, then the total mechanical energy of the system is:
View Solution
The kinetic energy of a body is increased by 21%. The percentage increase in the magnitude of its linear momentum is:
View Solution
A tennis ball of mass 50g thrown vertically up at a speed of 25 m s\(^{-1}\) reaches a maximum height of 25 m. The work done by the resistance forces on the ball is:
View Solution
The radius of gyration of a circular disc of radius \( R \), rotating about its diameter is:
View Solution
For a smoothly running analog clock, the angular velocity of its second hand in rad s\(^{-1}\) is:
View Solution
If the acceleration due to gravity on the surface of a planet is 2.5 times that on Earth and radius, 10 times that of the Earth, then the ratio of the escape velocity on the surface of a planet to that on Earth is:
View Solution
The time period of revolution of a planet around the sun in an elliptical orbit of semi-major axis \( a \) is \( T \). Then
View Solution
In an incompressible liquid flow, mass conservation leads to:
View Solution
The maximum velocity of a fluid in a tube for which the flow remains streamlined is called its:
View Solution
Coefficient of linear expansion of aluminum is \( 2.5 \times 10^{-5} \, K^{-1} \). Its coefficient of volume expansion in \( K^{-1} \) is:
View Solution
The efficiency of a Carnot engine operating between steam point and ice point is:
View Solution
The type of processes represented by the curves X and Y are:
View Solution
Two similar metallic rods of the same length \( l \) and area of cross section \( A \) are joined and maintained at temperatures \( T_1 \) and \( T_2 \) (\( T_1 > T_2 \)) at one of their ends as shown in the figure. If their thermal conductivities are \( K \) and \( \frac{K}{2} \) respectively. The temperature at the joining point in the steady state is:
View Solution
According to the equipartition principle, the energy contributed by each translational degree of freedom and rotational degree of freedom at a temperature \( T \) are respectively (\( k_B = Boltzmann constant \)):
View Solution
The kinetic energy of 3 moles of a diatomic gas molecules in a container at a temperature \( T \) is same as that of kinetic energy of \( n \) moles of monoatomic gas molecules in another container at the same temperature \( T \). The value of \( n \) is:
View Solution
A string of length \( L \) is fixed at both ends and vibrates in its fundamental mode. If the speed of waves on the string is \( v \), then the angular wave number of the standing wave is:
View Solution
Ratio between the frequencies of the third harmonics in the closed organ pipe and open organ pipe of the same length is:
View Solution
A tuning fork vibrating at 300 Hz, initially in air, is then placed in a trough of water. The ratio of the wavelength of the sound waves produced in air to that in water is (Given that the velocity of sound in water and in air at that place are 1500 m/s and 350 m/s respectively):
View Solution
The ratio of the magnitudes of electrostatic force between an electron and a proton separated by a distance \( r \) to that between a proton and an alpha particle separated by the same distance \( r \) is:
View Solution
The electric field due to an infinitely long thin wire with linear charge density \( \lambda \) at a radial distance \( r \) is proportional to:
View Solution
A spherical metal shell \( A \) of radius \( R_A \) and a solid metal sphere \( B \) of radius \( R_B \) (\( R_B < R_A \)) are kept far apart and each is given charge \( +Q \). If they are connected by a thin metal wire and \( Q_A \) and \( Q_B \) are the charge on \( A \) and \( B \), respectively, then:
View Solution
If the number of electron-hole pairs per cm\(^3\) of an intrinsic Si wafer at temperature 300 K is \(1.1 \times 10^{10}\) and the mobilities of electrons and holes at 300 K are 1500 and 500 cm\(^2\) per volt, second, respectively, then the conductivity of the Si wafer at this temperature (in \(\mu\)mho cm\(^{-1}\)) is nearly:
View Solution
Magnitude of drift velocity per unit electric field is known as:
View Solution
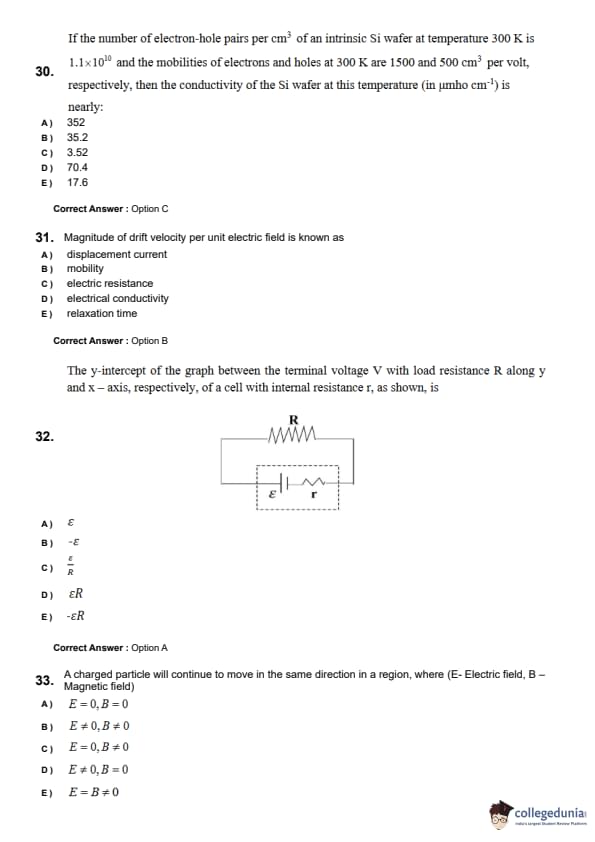
The y-intercept of the graph between the terminal voltage \(V\) with load resistance \(R\) along \(y\) and \(x\) – axis, respectively, of a cell with internal resistance \(r\), as shown, is:
View Solution
A charged particle will continue to move in the same direction in a region, where \(E\) - Electric field, \(B\) - Magnetic field:
View Solution
When an \( \alpha \) particle and a proton are projected into a perpendicular uniform magnetic field, they describe circular paths of the same radius. The ratio of their respective velocities is:
View Solution
An electric appliance draws 3A current from a 200 V, 50 Hz power supply. The amplitude of the supply voltage is nearly:
View Solution
The oscillating magnetic field in a plane electromagnetic wave is given by \(B_y = (8 \times 10^{-6}) \sin[2 \pi \times 10^{11} t + 200 \pi x] \) tesla. Then the wavelength of the electromagnetic wave (in cm) is:
View Solution
A path length of 1m in air is equal to a path length of \(x\) m in a medium of refractive index 1.5. Then the value of \(x\) (in meters) is:
View Solution
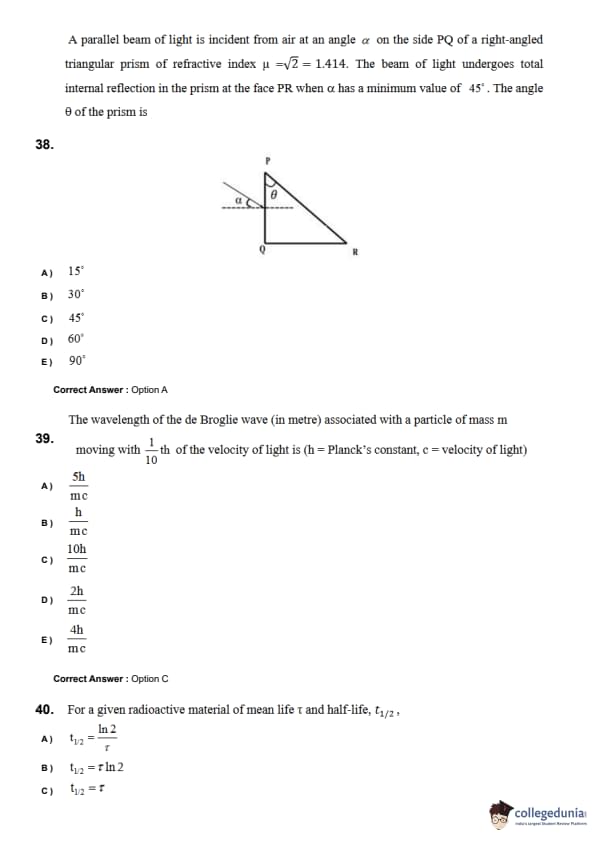
A parallel beam of light is incident from air at an angle \(\alpha\) on the side PQ of a right-angled triangular prism of refractive index \(\mu = \sqrt{2} \approx 1.414\). The beam of light undergoes total internal reflection in the prism at the face PR when \(\alpha\) has a minimum value of 45\(^{\circ}\). The angle \(\theta\) of the prism is:
View Solution
The wavelength of the de Broglie wave (in meter) associated with a particle of mass \(m\) moving with \(\frac{1}{10}\) of the velocity of light is (h = Planck's constant, c = velocity of light):
View Solution
For a given radioactive material of mean life \(\tau\) and half-life \(t_{1/2}\), the relationship between \(t_{1/2}\) and \(\tau\) is:
View Solution
The constancy of the binding energy per nucleon in medium-sized nuclei is due to:
View Solution
In a radioactive decay, the fraction of the number of atoms left undecayed after time \( t \) is:
View Solution
In the electron emission process, \( _{Z}^{A}X \rightarrow _{Z+1}^{A}Y + e^{-} + \bar{\nu} \), the particle \( q \) emitted along with the electron is:
View Solution
The current flowing from p to n side in a pn junction diode irrespective of biasing is termed:
View Solution
The energy required by the electron to cross the forbidden band for Germanium is:
View Solution
The molarity of sodium hydroxide in the solution prepared by dissolving 6 g in 600 mL of water is (molar mass of NaOH = 40 g mol\(^{-1}\)):
View Solution
The volume of ethanol required to prepare 3 L of 0.25 M aqueous solution is (density of ethanol = 0.36 kg L\(^{-1}\), molar mass = 60 g mol\(^{-1}\)):
View Solution
Which of the following statement is incorrect about Bohr's model of the atom?
View Solution
The decreasing order of first ionisation enthalpy of the following elements is:
View Solution
The hybridisation involved in the metal atom of \([CrF_6]^{3-}\) is:
View Solution
The valence electron MO configuration of \( C_2 \) (atomic number of C = 6) molecule is:
View Solution
Which of the following is used as anode in mercury cell?
View Solution
Which of the following is true for a reaction that is spontaneous only at high temperature?
View Solution
In a process, 600 J of heat is absorbed by a system and 375 J of work is done by the system. The change in internal energy of the process is:
View Solution
The value of \( K_c \) for the equilibrium reaction \[ 2 NO_2(g) \rightleftharpoons N_2O_4(g) \]
is \(2 \times 10^{-40} \, mol^{-1} \, dm^3\) at 298 K. If the equilibrium concentration of \(NO_2\) is \(2 \times 10^{-2}\) M, the concentration of \(N_2O_4\) is:
View Solution
The quantity of electricity required to produce 18 g of Al from molten Al\(_2\)O\(_3\) is (Atomic mass of Al = 27):
View Solution
The average oxidation state of sulphur in the tetrathionate ion is:
View Solution
The mass percentage of glucose in acetonitrile when 6 g of glucose is dissolved in 294 g of acetonitrile is:
View Solution
The rate constant of a first order reaction is \(4.606 \times 10^{-3} \, s^{-1}\). The time taken to reduce 20 g of reactant into 2 g is:
View Solution
The rate law for the reaction, A + B → Product, is:
\[ rate = [A][B]^{3/2} \]
The total order of the reaction is:
View Solution
Which of the following mixture forms azeotrope?
View Solution
A coordination compound of cobalt acts as an antipericious anemia factor is:
View Solution
The type of d-d transition of the electron occurs in \([Ti(H_2O)_6]^{3+}\) is:
View Solution
The increasing order of field strength of ligands in the spectrochemical series is:
View Solution
The reaction, \( 2I^- + S_2O_8^{2-} \rightarrow I_2 + 2SO_4^{2-} \), is catalysed by:
View Solution
Which of the following is used in the treatment of lead poisoning?
View Solution
The increasing order of acid strength of the following carboxylic acids is:
View Solution
The decreasing order of stability of the following carbocations is:
View Solution
The number of unpaired electrons in \([CoF_6]^{3-}\) is:
View Solution
One mole of an alkene on ozonolysis gives a mixture of one mole pentan-3-one and one mole methanal. The alkene is:
View Solution
A tertiary alkyl halide (X), C\(_4\)H\(_9\)Br, reacted with alc.KOH to give compound (Y). Compound (Y) reacted with HBr in presence of peroxide to give compound (Z). The compounds (Y) and (Z) are respectively:
View Solution
The major products formed when one mole of \( CH_3CH_2CH(CH_3)CH_2OCH_2CH_3 \) is treated with one mole of HI are:
View Solution
The reagent used for the conversion of but-2-ene to ethanol is:
View Solution
Which of the following is used as insect attractant?
View Solution
Lactose is composed of:
View Solution
If A and B are two sets, such that A has 20 elements, \( A \cup B \) has 32 elements, and \( A \cap B \) has 10 elements, the number of elements in the set B is:
View Solution
Let a relation \( R \) on the set of natural numbers be defined by \( (x, y) \in R \) if and only if \( x^2 - 4xy + 3y^2 = 0 \) for all \( x, y \in \mathbb{N} \). Then the relation is:
View Solution
If \( f(x) = \begin{cases} x^2 & for x < 0
5x - 3 & for 0 \leq x \leq 2
x^2 + 1 & for x > 2 \end{cases} \), then the positive value of \( x \) for which \( f(x) = 2 \) is:
View Solution
Let \( X \) and \( Y \) be subsets of \( \mathbb{R} \). If \( f : X \rightarrow Y \) given by \( f(x) = -8(x + 5)^2 \) is one-to-one, then the codomain \( Y \) is:
View Solution
Let \( z \) be a complex number satisfying \( |z + 16| = 4|z + 1| \). Then:
View Solution
If \( 2z = 7 + i\sqrt{3} \), then the value of \( z^2 - 7z + 4 \) is:
View Solution
If \( \left( \frac{1 - i}{1 + i} \right)^{10} = a + ib \), then the values of \( a \) and \( b \) are, respectively:
View Solution
If \( z_1 \) and \( z_2 \) are two complex numbers with \( |z_1| = 1 \), then \( \left| \frac{z_1 - z_2}{1 - z_1 \overline{z_2}} \right| \) is equal to:
View Solution
The second term of a G.P. is 4, then the product of the first three terms is:
View Solution
The common ratio of a G.P. is \( \frac{1}{2} \). If the product of the first three terms is 64, then the sum of the first 10 terms is:
View Solution
The numbers \( a, b, c, d \) are in G.P. with common ratio \( r \). If \( \frac{1}{a^3 + b^3} + \frac{1}{b^3 + c^3} + \frac{1}{c^3 + d^3} \) are also in G.P., then the common ratio is:
View Solution
The minimum value of \( f(x) = 7x^4 + 28x^3 + 31 \) is:
View Solution
Evaluate \( \binom{10}{1} + \binom{10}{2} + \dots + \binom{10}{10} \):
View Solution
The coefficient of \( x^3 \) in the binomial expansion of \( \left( \frac{1}{\sqrt{x}} - x \right)^6 \) is:
View Solution
If \( _nP_r = 480 \) and \( _nC_r = 20 \), then the value of \( r \) is equal to:
View Solution
The constant term in the expansion of \( \left( x^3 + \frac{1}{x^2} \right)^{10} \) is:
View Solution
If \[ \begin{bmatrix} 3 & 4
5 & x \end{bmatrix} + \begin{bmatrix} 1 & y
0 & 1 \end{bmatrix} = \begin{bmatrix} 7 & 0
10 & 5 \end{bmatrix}, \]
then the value of \( x - y \) is:
View Solution
If \( B = \begin{bmatrix} 1 & \alpha & 3
1 & 3 & 3
2 & 4 & 4 \end{bmatrix} \) is the adjoint of a \( 3 \times 3 \) matrix \( A \) and \( |A| = 4 \), then the value of \( \alpha \) is:
View Solution
If the points \( (2, -3), (\lambda, -1) \) and \( (0, 4) \) are collinear, then the value of \( \lambda \) is equal to:
View Solution
The solution set for the inequalities \( -5 \leq \frac{2 - 3x}{4} \leq 9 \) is:
View Solution
If the determinant of the matrix \( \begin{bmatrix} |x| & 1 & 2
4 & 1 & x
1 & -1 & 3 \end{bmatrix} \) equals -10, then the values of \( x \) are:
View Solution
Let \( A = (a_{ij}) \) be a square matrix of order 3 and let \( M_{ij} \) be the minors of \( a_{ij} \). If \( M_{11} = -40, M_{12} = -10, M_{13} = 35 \), and \( a_{11} = 1, a_{12} = 3, a_{13} = -2 \), then the value of \( |A| \) is equal to:
View Solution
If \( \frac{\sec^2 15^\circ - 1}{\sec^2 15^\circ} \) equals:
View Solution
The value of \( \sin^2 \left( \frac{3\pi}{8} \right) + \sin^2 \left( \frac{7\pi}{8} \right) \) is:
View Solution
If \( \sin \theta = \frac{b}{a} \), then \( \frac{\sqrt{a+b}} {\sqrt{a-b}} + \frac{\sqrt{a-b}} {\sqrt{a+b}} \) is equal to:
View Solution
The period of \( 2 \sin 4x \cos 4x \) is:
View Solution
The domain of the function \( f(x) = \frac{\sin^{-1} \left( x-3 \right)}{\sqrt{9 - x^2}} \) is:
View Solution
If \( \alpha = \tan^2 x + \cot^2 x \), where \( x \in \left( 0, \frac{\pi}{2} \right) \), then \( \alpha \) lies in the interval:
View Solution
The value of \( \tan \left[ \tan^{-1} \left( \frac{3}{4} \right) + \tan^{-1} \left( \frac{2}{3} \right) \right] \) is:
View Solution
If \( 3 \sin \theta + 5 \cos \theta = 5 \), then the value of \( 5 \sin \theta - 3 \cos \theta \) is:
View Solution
Evaluate \( \cos \left( \cot^{-1} \left( \frac{7}{24} \right) \right) \):
View Solution
If \( \cos \theta = \frac{2 \cos \alpha + 1}{2 + \cos \alpha} \), then \( \tan^2 \left( \frac{\theta}{2} \right) \) is equal to:
View Solution
If a vector makes angles \( \frac{\pi}{3}, \frac{\pi}{4} \) and \( \gamma \) with \( \hat{i}, \hat{j} \), and \( \hat{k} \), respectively, where \( \gamma \in \left( \frac{\pi}{2}, \pi \right) \), then the angle \( \gamma \) is:
View Solution
Let \( \mathbf{u}, \mathbf{v}, \mathbf{w} \) be vectors such that \( \mathbf{u} + \mathbf{v} + \mathbf{w} = \mathbf{0} \). If \( |\mathbf{u}| = 3 \), \( |\mathbf{v}| = 4 \), and \( |\mathbf{w}| = 5 \), then \( \mathbf{u} \cdot \mathbf{v} + \mathbf{w} \cdot \mathbf{u} \) is:
View Solution
Let \( \vec{a} = \hat{i} - \hat{j} \), \( \vec{b} = \hat{j} - \hat{k} \), and \( \vec{c} = \hat{k} - \hat{i} \), then the value of \( \vec{b} \cdot (\vec{a} + \vec{c}) \) is:
View Solution
Let \( \vec{a}, \vec{b}, \vec{c} \) be three vectors with magnitudes 4, 4, and 2, respectively. If \( \vec{a} \) is perpendicular to \( (\vec{b} + \vec{c}) \), \( \vec{b} \) is perpendicular to \( (\vec{c} + \vec{a}) \), and \( \vec{c} \) is perpendicular to \( (\vec{a} + \vec{b}) \), then the value of \( |\vec{a} + \vec{b} + \vec{c}| \) is:
View Solution
If two vectors \( \vec{a} = \cos \alpha \hat{i} + \sin \alpha \hat{j} + \sin \frac{\alpha}{2} \hat{k} \) and \( \vec{b} = \sin \alpha \hat{i} - \cos \alpha \hat{j} + \cos \frac{\alpha}{2} \hat{k} \) are perpendicular, then the values of \( \alpha \) are:
View Solution
If one end of a diameter of the circle \( x^2 + y^2 - 4x - 6y + 11 = 0 \) is \( (3, 4) \), then the coordinate of the other end of the diameter is:
View Solution
If the focus of a parabola is \( (0, -3) \) and its directrix is \( y = 3 \), then its equation is:
View Solution
The length of the minor axis of the ellipse with foci \( (\pm 2, 0) \) and eccentricity \( \frac{1}{3} \) is:
View Solution
The equation of the line passing through the point \( (1, 2) \) and perpendicular to the line \( x + y + 1 = 0 \) is:
View Solution
The line \( \frac{x}{5} + \frac{y}{b} = 1 \) passes through the point \( (13, 32) \) and is parallel to the line \( \frac{x}{c} + \frac{y}{3} = 1 \). Then the values of \( b \) and \( c \) are, respectively:
View Solution
A ray of light passing through the point \( (1, 2) \) is reflected on the \( x \)-axis at a point \( P \) and passes through the point \( (5, 6) \). Then the abscissa of the point \( P \) is:
View Solution
If the straight line \( \frac{x - a}{1} = \frac{y - b}{2} = \frac{z - 3}{-1} \) passes through \( (-1, 3, 2) \), then the values of \( a \) and \( b \) are, respectively:
View Solution
The lines \( \frac{x + 3}{-2} = \frac{y}{1} = \frac{z - 4}{3} \) and \( \frac{x - 1}{\mu} = \frac{y - 1}{\mu + 1} = \frac{z}{\mu + 2} \) are perpendicular to each other. Then the value of \( \mu \) is:
View Solution
If the straight lines \( \frac{x - 3}{2} = \frac{y - 4}{3} = \frac{z - 6}{-1} \) and \( \frac{x - 2}{a} = \frac{y + 3}{b} = \frac{z + 4}{-1} \) are parallel, then \( a^2 + b^2 \) is:
View Solution
The angle between the lines \( \frac{x}{1} = \frac{y}{1} = \frac{z}{1} \) and \( \frac{x}{0} = \frac{y}{1} = \frac{z}{-1} \) is:
View Solution
If three distinct numbers are chosen randomly from the first 50 natural numbers, then the probability that all of them are divisible by 2 and 3 is:
View Solution
If \( \frac{1 + 3p}{4}, \frac{1 - p}{3}, \frac{1 - 3p}{2} \) are the probabilities of three mutually exclusive and exhaustive events, then the value of \( p \) is:
View Solution
The mean deviation of the numbers 3, 10, 10, 4, 7, 10 and 5 from the mean is:
View Solution
If \( g(x) = -\sqrt{25 - x^2} \), then \( g'(1) \) is:
View Solution
Evaluate \( \lim_{x \to 0} \frac{\sin 2x + \sin 5x}{\sin 4x + \sin 6x} \):
View Solution
If \( f(x) = \left\{ \begin{array}{ll} mx + 1, & when x \leq \frac{\pi}{2}
\sin x + n, & when x > \frac{\pi}{2} \end{array} \right. \) is continuous at \( x = \frac{\pi}{2} \), then the values of \( m \) and \( n \) are:
View Solution
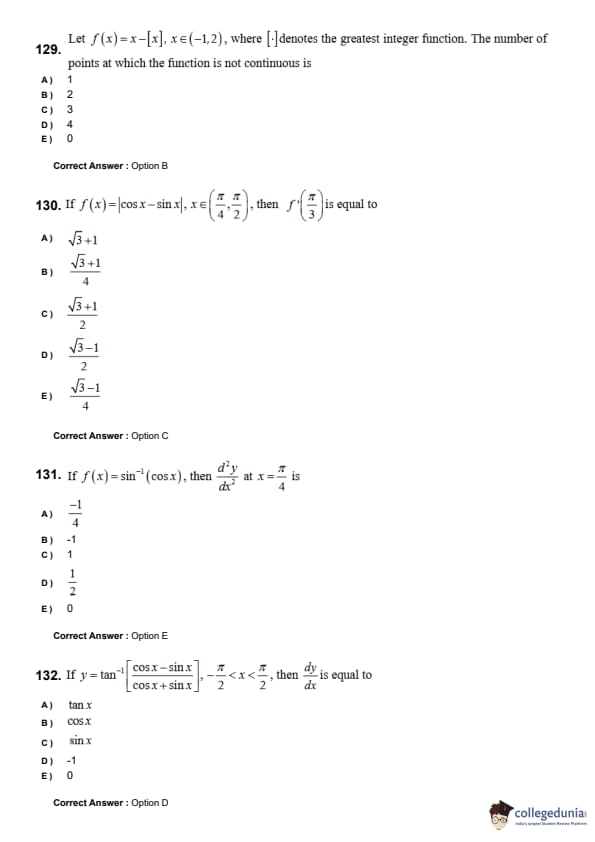
Let \( f(x) = x - \lfloor x \rfloor \), where \( \lfloor \cdot \rfloor \) denotes the greatest integer function and \( x \in (-1, 2) \). The number of points at which the function is not continuous is:
View Solution
If \( f(x) = \cos x - \sin x \), and \( x \in \left( \frac{\pi}{4}, \frac{\pi}{2} \right) \), then \( f' \left( \frac{\pi}{3} \right) \) is equal to:
View Solution
If \( f(x) = \sin^{-1}(\cos x) \), then \( \frac{d^2 y}{dx^2} \) at \( x = \frac{\pi}{4} \) is:
View Solution
If \( y = \tan^{-1} \left( \frac{\cos x - \sin x}{\cos x + \sin x} \right) \), \( \frac{-\pi}{2} < x < \frac{\pi}{2} \), then \( \frac{dy}{dx} \) is:
View Solution
If \( y = \frac{x^2}{x - 1} \), then \( \frac{dy}{dx} \) at \( x = -1 \) is:
View Solution
The function \( f(x) = 2x^3 + 9x^2 + 12x - 1 \) is decreasing in the interval:
View Solution
The maximum value of \( y = 12 - |x - 12| \) in the range \( -11 \leq x \leq 11 \) is:
View Solution
The limit \( \lim_{x \to 10} \frac{x - 10}{\sqrt{x + 6} - 4} \) is equal to:
View Solution
The integral \( \int \frac{dx}{1 + e^x} \) is:
View Solution
Evaluate \( \int x \cos x \, dx \):
View Solution
Evaluate \( \int x e^{x^2} \, dx \):
View Solution
If \[ \int \frac{dx}{\sqrt{16 - 9x^2}} = A \sin^{-1}(Bx) + C, where C is an arbitrary constant, then A + B = \]
View Solution
Evaluate \( \int \frac{dx}{x^2 (x^4 + 1)^{3/4}} \):
View Solution
Evaluate \( \int \frac{e^{6 \log x} - e^{5 \log x}}{e^{4 \log x} - e^{3 \log x}} \, dx \):
View Solution
Evaluate \( \int_0^1 \log \left( \frac{1}{x - 1} \right) \, dx \):
View Solution
Evaluate \( \int_{-\pi/2}^{\pi/2} \sin^9 x \cos^2 x \, dx \):
View Solution
Find the area bounded by the curves \( y = 2x \) and \( y = x^2 \):
View Solution
Find the area of the smaller segment cut-off from the circle \( x^2 + y^2 = 25 \) by \( x = 3 \):
View Solution
The differential equation \( \frac{dy}{dx} + x = A \) (where A is constant) represents:
View Solution
The general solution of \( \frac{dy}{dx} + y = 5 \) is:
View Solution
The degree of the differential equation \( (y^m)^2 + (\sin y')^4 + y = 0 \) is:
View Solution
Given the Linear Programming Problem:
Maximize \( z = 11x + 7y \)
subject to the constraints: \( x \leq 3 \), \( y \leq 2 \), \( x, y \geq 0 \).
Then the optimal solution of the problem is:
View Solution


































Comments